Home

What is Solubility?

The solubility of a solute in a solvent is the amount of the solute in moles that will saturate 1 dm³ (or 1000 g) of the solvent (water) at a particular temperature.

1. What is the solubility of potassium trioxonitrate(V), KNO₃ at 20^oC if 8.60 g of it is dissolved completely in 250 cm³ of water. (K=39, N=14, O=16).

Solution:

solubility = number of moles/volume (dm³)

number of moles of KNO₃ = mass/molar mass

                         = 8.60/101 = 0.0851 mole

solubility = 0.0851 mole/0.25 dm³

             = 0.34 mole/dm³

Note: solubility is same as molarity.

2. The solubility of potassium trioxonitrate(V) at 15^oC is 25.8 g in 100 g of water. Calculate the mass of the salt in 25 g of saturated solution at 15^oC.

Solution:

Weight (or mass) of saturated solution at 15^oC = mass of salt + mass of water = 25.8 g + 100 g = 125.8 g \ 125.8 g of saturated solution contains 25.8 g of salt.

Hence, 25 g of saturated solution will contain 25.8 25 g of salt 125.8 Note: when a solution is saturated, the weight (or mass) of the solution is the sum of the masses of the salt and the solvent (i.e. water).

The solubility of solids in liquids depends on:

- Nature of solutes and solvents – polar solutes dissolve more in polar solvents than in non-polar solvents. Also, non-polar solutes dissolve more in non-polar solvents. Example, NaCl (polar solute ) dissolves readily in water (polar solvent) than in gasoline (non polar solvent); naphthalene (non-polar solute) dissolves more in gasoline (non polar solvent) than in water (polar solvent).

- Temperature - the higher the temperature, the higher the solubility. This is because the rate of separation or dissociation of solute particles and the rate of separation of solvent particles increase with rise in temperature and vice versa.

Note: The dissolution of a solid in a liquid goes through:

1. The separation of solute particles into gaseous ions - heat energy is required (crystal or lattice energy).

2. The separation of solvent particles - heat energy is required.

3. The hydration or solvation of the gaseous ions by water– heat energy is released (hydration energy).

The solubility of gases in liquids depends on:

- Nature of gas and liquid – similarity in chemical properties between gas and solvent increases solubility. Example, organic gases (e.g. methane) dissolve readily in organic solvents (e.g. benzene). Inorganic gases dissolve more in inorganic solvents. Example, ammonia dissolves readily in water - this is further aided by the formation of hydrogen bonding between ammonia and water.

Also, hydrochloric acid dissolves readily in water (hydrogen bonding also aid the dissolution).

- temperature - notice that at constant pressure, the solubility of a gas decreases with increase in temperature (the dissolution of gases in liquids is generally an exothermic reaction, hence, boiling may expel the gas from its solution).

- pressure - at constant temperature, the solubility of a gas increases with rise in pressure, and vice versa. The solubility of a gas in a liquid is measured in terms of absorption coefficient – the higher the absorption coefficient, the greater the solubility, and vice versa.

The solubility of solids in liquids generally vary with temperature. Most substances show steady increases in solubility with rise in temperature. However, few solute, like Ca(OH)₂, CaSO₄ and Na₂SO₄ show initial increase in solubility with rise in temperature, but at high temperatures, their solubilities begin to decrease.

A solubility curve is obtained when the solubilities of a substance determined at various temperatures are plotted against temperature. From the curve, certain deductions can easily be made. Below are the solubility curves of three compounds: X (molar mass 74.5 g), Y (molar mass 122.5 g) and Z (molar mass 58.5 g). The following deductions can be made from them:

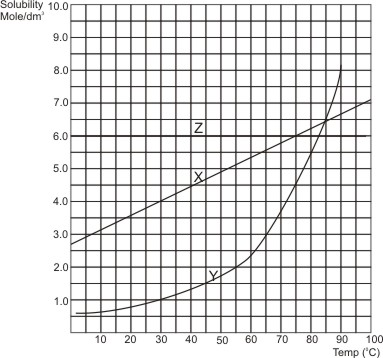
(i). The solubility of a compound at any required temperature. To obtain the solubility corresponding to a temperature, trace vertically upwards from the temperature point to touch the curve. At the point it touches the curve, trace horizontally to the solubility axis. This gives the solubility (i.e., the amount of the substance in moles/dm³ that can dissolve at that particular temperature).

Example, at 30^oC, the solubility of X is 4.0 moles/dm³, that of Y is 1.0 mole/dm³, while that of Z is 6.0 mole/dm³.

(ii). The quantity of a compound precipitated or crystallized from the solution when temperature drops. Notice that when the temperature of a saturated solution falls, part of the solute becomes insoluble and therefore goes out of solution as precipitates or crystals.

Example, when the solution is cooled from 70⁰C to 30⁰C: at 70⁰C, 5.8 moles of X are soluble in 1 dm³ of solution. at 30⁰C, 4.0 moles of X are soluble in 1 dm³ of solution.

Therefore, cooling from 70⁰C to 30⁰C, 5.8 - 4.0 = 1.8 mole of X will leave 1 dm³ of the solution. Converting to mass, we have: mass = number of moles x molar mass = 1.8 x 74.5 = 134.1 g

Notice that the solubility of Z is not affected by change in temperature (i.e., solubility is constant), example of substances with this kind of solubility curve is NaCl.

Question: from the above solubility curves, deduce which compound is the impurity and the percentage impurity, when temperature is reduced from 70⁰C to 30⁰C.

Solution: we have already shown that the quantity of X leaving the solution is 1.8 mole.

For Y: at 70⁰C, 3.6 moles of Y are in solution. At 30⁰C, 1.0 mole of Y is in solution.

Therefore, cooling from 70⁰C to 30⁰C, 3.6 - 1.0 = 2.6 moles of Y leave the solution.

From the result, X is the impurity since its quantity (in moles) that is insoluble is smaller than that of Y.

Percentage impurity = number of moles of X insoluble/(number of moles of X + number of moles of Y) insoluble x 100

                              = 1.8/(1.8+2.6) x 100 = 40.9%

(iii). The points where the solubilities of two or more compounds are equal. Notice that this point is where their curves meet.

Example, from the above curves, the solubilities of X and Y are equal (i.e. 6.4 moles/dm³) at 84^oC.